Question

A spherical hot-air balloon is initially filled with air at 120 kPa and 20°C with an initial diameter of 5 m. Air enters this balloon at 120 kPa and 20°C with a velocity of 3 m/s through a 1-m-diameter opening. How many minutes will it take to inflate this balloon to a 17-m diameter when the pres-sure and temperature of the air in the balloon remain the same as the air entering the balloon?

Answer 1

time is 17.43 min

Step-by-step explanation:

given data

initial diameter = 5 m

velocity = 3 m/s

final diameter = 17 m

solution

we will apply here change in change in volume equation that is express as

ΔV =
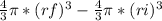`(4)/(3) \pi * (rf)^3 - (4)/(3) \pi * (ri)^3` .............1

here ΔV is change in volume and rf is final radius and ri is initial radius

ΔV =
`(4)/(3) \pi * (8.5)^3 - (4)/(3) \pi * (2.5)^3`

ΔV = 2507 m³

so

Q = velocity × Area

Q = 3 × π ×(0.5)² = 2.356 m³/s

and

change in time is express as

Δt =
`(\Delta V)/(Q)`

Δt =
`(2507)/(2.356)`

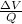
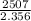
Δt = 1046 sec

so change in time is 17.43 min