Answer: 0.5363
Explanation:
Given : Population mean :
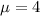
and standard deviation :

sample size : n= 100
Let x be the random variable that represents the thickness of sheet.
Since the probability for each element in a Simple random sample is equal.
∴ Using formula
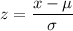 ,
,
The z-value corresponds to x=4.1
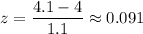
The probability that the sample mean would be greater than 139.7 millimeters will be :-
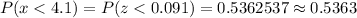
Hence, the required probability : 0.5363