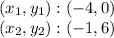Question

1) Solve the following system of equations algebraically. Give your solution as two

ordered pair (x, y)
y=x2 + 7x + 12,
y = 2x + 8

Answer 1

For this case we have the following system of equations:

`y = x ^ 2 + 7x + 12\\y = 2x + 8`

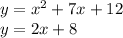
Equating both equations we have:

`x ^ 2 + 7x + 12 = 2x + 8\\x ^ 2 + 7x-2x + 12-8 = 0\\x ^ 2 + 5x + 4 = 0`

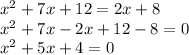
We must find the solutions, for this we factor. We look for two numbers that, when multiplied, result in 4 and when added, result in 5. These numbers are 4 and 1:

`4 + 1 = 5\\4 * 1 = 4`

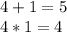
Then, the factorized equation is of the form:

`(x + 4) (x + 1) = 0`

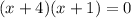
Thus, the solutions are:

`x_ {1} = - 4\\x_ {2} = - 1`

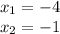
We look for solutions for the variable "y":

`y_ {1} = 2 (-4) + 8 = -8 + 8 = 0\\y_ {2} = 2 (-1) + 8 = -2 + 8 = 6`

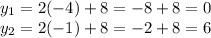
Thus, the system solutions are given by:
`(x_ {1}, y_ {1}): (- 4,0)\\(x_ {2}, y_ {2}): (- 1,6)`

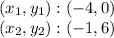
ANswer:

`(x_ {1}, y_ {1}): (- 4,0)\\(x_ {2}, y_ {2}): (- 1,6)`