2 Answers

Mqchen · Answer 1 · 2020-05-06T15:49:39+0000

The value of x in the equation
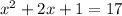x^(2)+2 x+1=17 is x = -5.123 or 3.123

Solution:

There are two ways to solve this equation.

We can either factorize it or use the quadratic equation. For factorizing it, it has to satisfy certain conditions

The condition is
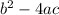b^(2)-4 a c should be a perfect square otherwise the equation is not factorable.

We have to rearrange the given equation to find values of a, b and c.

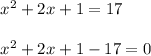
$\begin{array}{l}{x^(2)+2 x+1=17} \\\\ {x^(2)+2 x+1-17=0}\end{array}$

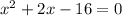x^(2)+2 x-16=0 ----- eqn 1

From eqn 1:

a = 1

b = 2

c = -16

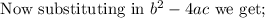
$\text { Now substituting in } b^(2)-4 a c \text { we get; }$

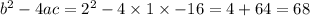
b^(2)-4 a c=2^(2)-4 * 1 *-16=4+64=68

Which is not a perfect square.

Hence we have to use the quadratic equation formula, which is:

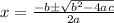
$x=\frac{-b \pm \sqrt{b^(2)-4 a c}}{2 a}$

By substituting the values of a, b and in the quadratic equations. We get;

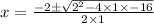
$x=\frac{-2 \pm \sqrt{2^(2)-4 * 1 *-16}}{2 * 1}$

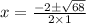
$x=(-2 \pm √(68))/(2 * 1)$

The two roots of x are:

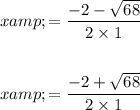
$\begin{aligned} x &=(-2-√(68))/(2 * 1) \\\\ x &=(-2+√(68))/(2 * 1) \end{aligned}$

On solving both the equations we will get the roots of the given equation, which are:

x = -5.123 or 3.123

Thus the value of "x" is found out

Please log in or register to add a comment.