Question

#16: How do I get the solution to this problem. I know the answer is ~4.2, but I wanna know how I get there

Answer 1

log₃(108) ≈ 4.26186

Explanation:

For logarithms to bases other than e or 10, the change of base formula is used:

`\log_b{(x)}=\frac{\log{(x)}}{\log{(b)}}`

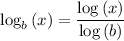
Your logarithm is ...

`\log_3{108}=\frac{\log{108}}{\log{3}}\approx(2.033424)/(0.4771213)\approx 4.26186`

_____

Comment on log bases

e (≈ 2.7182818284590...) is the (irrational) base of natural logarithms. Most modern scientific calculators will give logarithms to this base, or to base 10.

Many math formulas use natural logs by default. Consequently, you need to be a little careful with certain math tables and calculus formulas when "log" shows up. It may mean the natural log, not the base-10 log usually associated with that function name.

Base 10 logarithms tend to be more useful for numerical calculations, as the characteristic (integer part) translates directly to order of magnitude or the exponent in scientific notation.

If you want logarithms in other bases, a specialized calculator or the change of base formula must be used. The "log" in the change of base formula can be a logarithm to any base, as long as the numerator and denominator logs are to the same base.