Answer: 0.7745
Explanation:
Given : The length of time it takes a college student to find a parking spot in the library parking lot follows a normal distribution with
 minutes and
minutes and
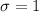 minute.
minute.
Let x represents the time to find a parking spot in the library parking lot.
Since ,

z-score corresponds x = 2 ,
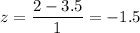
z-score corresponds x = 4.5 ,
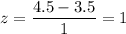
Required probability :
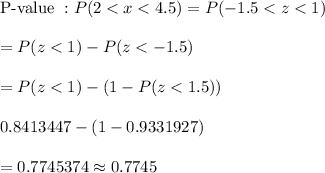
[using z-value table.]
Hence, the probability that a randomly selected college student will take between 2 and 4.5 minutes to find a parking spot in the library parking lot, (rounding to four decimal place )= 0.7745