Answer:
v = √(2G M /
 )
)
Step-by-step explanation:
To find the escape velocity we can use the concept of conservation of mechanical energy, where we take at two points one on the surface of the asteroid and another distant point
Initial
Em₀ = K = ½ mv² - G m M /

Final
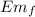 = U = - G m M /
= U = - G m M /

Where G is the universal gravitation constant value of 6.67 10⁻¹¹ Nm²/kg², M the mass of the asteroid and r is the distance from the stone to the asteroid
Em₀ =
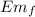
½ mv² - G m M /
 = - G m M /
= - G m M /

let's solve
½ v² = G M (1 /
 -1 /
-1 /
 )
)
The maximum distance value can be made infinite, so the last term becomes zero, so the escape velocity is
v = √(2G M /
 )
)
where we assume that we know the mass and radius of the asteroid