Question

An unknown immiscible liquid seeps into the bottom of an open oil tank. Some measurements indicate that the depth of the unknown liquid is 1.5 m and the depth of the oil(specificweight=8.5kN/m3)floating on top is 5.0 m. A pressure gage connected to the bottom of the tank reads 65 kPa. What is the specific gravity of the unknown liquid?

Answer 1

The specific gravity of the unkown liquid is 15.

Step-by-step explanation:

Gauge pressure, at the bottom of the tank in this case, can be calculated from

`P_(bot)=\gamma_(oil)h_1 + \gamma_(unk)h_2,`

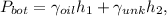
where
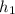`h_1` and
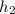`h_2` are the height of the column of oil and the unkown liquid, respectively. Writing for
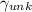`\gamma_(unk)`, we have

`\gamma_(unk) = (P_(bot) - \gamma_(oil)h_1)/(h_2) = (65(kN)/(m^2) - 8.5(kN)/(m^3)* 5m)/(1.5m) = \mathbf{15 (kN)/(m^3)}.`

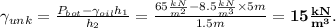
Relative to water, the unknow liquid specific weight is 15 times bigger, therefore this is its specific gravity as well.