Answer: 0.6381
Explanation:
Given : A manufacturer of industrial solvent guarantees its customers that each drum of solvent they ship out contains at least 100 lbs of solvent.
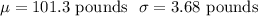
Let x be a random variable that represents e the amount of solvent in each drum.
Since ,

z-score corresponds x = 100 ,
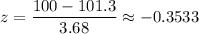
Required probability :
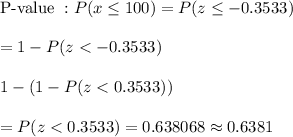
[using z-value table.]
Hence, the probability that a drum meets the guarantee = 0.6381