Question

Delicious Candy markets a two-pound box of assorted chocolates. Because of imperfec- tions in the candy making equipment, the actual weight of the chocolate has a uniform distribution ranging from 31 to 32.5 ounces. What is the probability that a box weighs less than 31.8 ounces?

Answer 1

The required probability is 0.533.

Explanation:

Consider the provided information.

The actual weight of the chocolate has a uniform distribution ranging from 31 to 32.5 ounces.

Let x is the random variable for the actual weight of chocolate.

According to PDF function.

`P(a\leq x\leq b)=\int\limits^b_a {f(x)} \, dx`

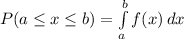
Where
`f(x)=\left\{\begin{matrix}(1)/(b-a) & a<x<b\\ 0 & otherwise \end{matrix}\right.`

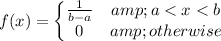
It is given that ranging from 31 to 32.5 ounces.

Substitute a=31 and b=32.5 in above function.

`f(x)=\left\{\begin{matrix}(1)/(32.5-31) & 31<x<32.5\\ 0 & otherwise \end{matrix}\right.`

`f(x)=\left\{\begin{matrix}(1)/(1.5) & 31<x<32.5\\ 0 & otherwise \end{matrix}\right.`

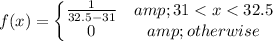
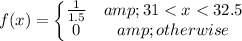
We need to find the probability that a box weighs less than 31.8 ounces

Now according to PDF:

`P(x<31.8)=\int\limits^(31.8)_(31) {(1)/(1.5) \, dx`

`P(x<31.8)=(1)/(1.5)[31.8-31]\\P(x<31.8)=(0.8)/(1.5)\\P(x<31.8)=0.533`

Hence, the required probability is 0.533.