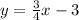2 Answers

Aei · Answer 1 · 2020-02-20T00:58:09+0000

Answer:

The equation of a line in slope-intercept from with an x-intercept of 4 and a y-intercept of -3 is
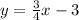
y=(3)/(4) x-3

Solution:

Given, a line in slope-intercept from with an x-intercept of 4 and a y-intercept of -3

We have to find the line equation with above given intercept values.

We know that, line equation in intercepts form is

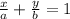
(x)/(a)+(y)/(b)=1

where a, b are x, y intercepts respectively.

$\text { Now, our required line is } (x)/(4)+(y)/(-3)=1 \rightarrow (x)/(4)-(y)/(3)=1$

Now our equation in slope intercept form will be
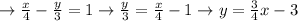
$\rightarrow (x)/(4)-(y)/(3)=1 \rightarrow (y)/(3)=(x)/(4)-1 \rightarrow y=(3)/(4) x-3$

So, we derived the equation in slope intercept form where slope =3/4 and intercept = -3

Hence, the slope intercept form the line is
y=(3)/(4) x-3

Please log in or register to add a comment.