Question

An adiabatic air compressor compresses 10 L/s of air at 120 kPa and 20°C to 1000 kPa and 300°C. Determine (a) the work required by the compressor, in kJ/kg, and (b) the power required to drive the air compressor, in kW. Air = 0.287 kPa m3 /kgK. cp,avg = 1.02 kJ/kgC

Answer 1

Answers:

a)
`290.875 kJ/kg`

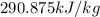
b)
`4.07 kW`

Step-by-step explanation:

a) Since air is considered an ideal gas, its gas constant
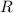`R` is established in a Thermodynamic table as
`R=0.287 (kPa m^(3))/(kg K)`.

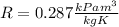On the other hand, we have to convert the temperatures from Celsius to Kelvin:

`T_(1)=20\°C + 273.15 K=293.15 K \approx 295 K`

`T_(2)=300\°C + 273.15 K=573.15 K \approx 580 K`

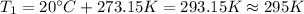
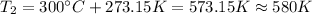
Then, we can find the enthalpy
`h` from another Thermodynamic table (A-17) with this given temperatures in Kelvin:

For
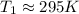`T_(1)\approx 295 K`,
`h_(1)=295.17 kJ/kg` (1)

For
`T_(2)\approx 580 K`,
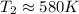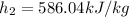`h_(2)=586.04 kJ/kg` (2)

Now, the work required by the compressor (the work input)
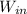`W_(in)` is given by the following equation for
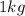`1 kg` of air (assuming there is only one inlet and one exit in this system, where the volume is controlled):

`W_(in)=\Delta h=h_(2)-h_(1)` (3)

`W_(in)=586.04 kJ/kg - 295.17 kJ/kg`

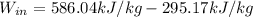
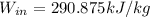`W_(in)=290.875 kJ/kg` (4) This is the work required by the air compressor

b) The power input
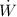`\dot{W}` is given by:

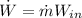`\dot{W}=\dot{m}W_(in)` (5)

Where
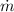`\dot{m}` is the mass flow rate at the inlet:

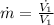`\dot{m}=\frac{\dot{V_(1)}}{V_(1)}` (6)

We already know the volume flow rate
`\dot{V_(1)}`:

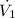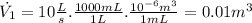`\dot{V_(1)}=10(L)/(s). (1000 mL)/(1 L) . (10^(-6) m^(3))/(1 mL)=0.01 m^(3)` (7)

The volume at the inlet
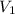`V_(1)` is given by the Ideal gas law equation:

`V_(1)=(R T_(1))/(P_(1))` (8)

`V_(1)=((0.287 (kPa m^(3))/(kg K))(295 K))/(120 kPa)` (9)

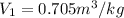`V_(1)=0.705 m^(3)/kg` (10)

Substituting (7) and (10) in (6):

`\dot{m}=(0.01 m^(3))/(0.705 m^(3)/kg)` (11)

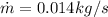`\dot{m}=0.014 kg/s` (12)

Substituting (12) in (5):

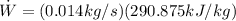`\dot{W}=(0.014 kg/s)(290.875 kJ/kg)` (5)

Finally:

`\dot{W}=4.07 kK/s = 4.07 kW`