Question

The pilot of a Coast Guard patrol aircraft on a search mission had just spotted a disabled fishing trawler and decided to go in for a closer look. Flying in a straight line at a constant altitude of 1000 ft and at a steady speed of 232 ft/sec, the aircraft passed directly over the trawler. How fast was the aircraft receding from the trawler when it was 1600 ft from the trawler? (Round your answer to one decimal places.)

Answer 1

`(dz)/(dt) =181.11\ ft/s`

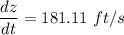
Step-by-step explanation:

given,

constant altitude of aircraft (y)= 1000 ft

steady speed of aircraft = 232 ft/sec

distance between aircraft and trawler(z) = 1600 ft

using Pythagoras theorem

z² = x² + y²

`x = √(1600^2-1000^2)`

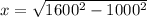
x = 1249 m

`(dy)/(dt) = 0`

`(dx)/(dt)= 232`

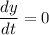
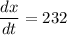
differentiating both side w.r.t to t

`2z (dz)/(dt) = 2x (dx)/(dt) + 2y(dy)/(dt)`

`z (dz)/(dt) = x (dx)/(dt)`

`(dz)/(dt) =(x)/(z) (dx)/(dt)`

`(dz)/(dt) =(1249)/(1600) * 232`

`(dz)/(dt) =181.11\ ft/s`

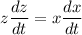
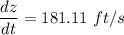
so the speed at which trawler is receding is 181.11 ft/s