Question

*Urgent*

Ben wants to buy licorice, jelly beans, or some of each for his party. The licorice costs $4 per pound, and the jelly beans cost $3 per pound. He will spend at least $11 but no more than $15. Which system of inequalities models the situation?

A. 4x+3y ≥ 11
4x+3y ≥ 15
X ≥0
Y ≥0

B. 4x +3y ≤ 11
4x + 3y ≥ 15
X ≥0
Y ≥0

C. 4x+3y ≤ 11
4x+3y ≤ 15
X ≥ 0
Y ≥ 0

D. 4x+3y ≥ 11
4x+3y ≤ 15
X ≥0
Y ≥0


(Picture will also be provided)

Answer 1

The anwser is D.

Explanation:

The equation must be greater or equal to eleven but less then or equal to 15.

Answer 2

Answer: D. 4x+3y ≥ 11

4x+3y ≤ 15

X ≥0

Y ≥0

Explanation:

Let x = Number of pounds of licorice.

y= Number of pounds of jelly beans .

Given : The licorice costs $4 per pound, and the jelly beans cost $3 per pound.

Cost of x licorice ( in dollars) = 4x

Cost of y jelly beans ( in dollars) = 3y

He will spend at least $11 but no more than $15.

i.e.
`4x+3y\geq11`

`4x+3y\leq15`

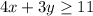
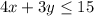
Here ,
`x\geq0 , y\geq0`

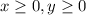
It means the required system of inequalities models the situation are:

`4x+3y\geq11`

`4x+3y\leq15`

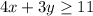
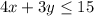
Here ,
`x\geq0 , y\geq0`

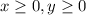
Thus , the option D is the correct answer.