Question

When a fighter pilot makes a very quick turn, he experiences a centripetal acceleration. When this acceleration is greater than about 5.5 × g the pilot will usually lose consciousness ("black out"). Consider a pilot flying at a speed of 890 m/s who wants to make a very sharp turn. What is the minimum radius of curvature that he can take without blacking out?

Answer 1

16.495 m

Step-by-step explanation:

a = Centripetal acceleration = 5.5 g

r = Radius of turn

v = Velocity of fighter jet = 890 m/s

g = Acceleration due to gravity = 9.81 m/s²

The direction of the velocity vector keeps constantly changing in centripetal acceleration

`a=(v^2)/(r)\\\Rightarrow r=(v^2)/(a)\\\Rightarrow r=(890)/(5.5* 9.81)\\\Rightarrow r=16.495\ m`

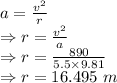
The minimum radius of curvature that he can take without blacking out is 16.495 m