Answer
given,
angular speed of the tub = 5 rev/s
time = 9 s
he tub slows to rest = 15.0 s
the angular acceleration
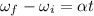
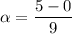
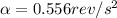
angular displacement
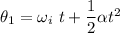
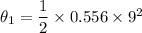
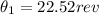
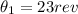
case 2
now,

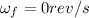
time = 15 s
the angular acceleration
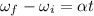
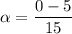
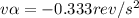
angular displacement
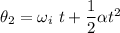
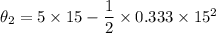

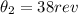
total revolution in 24 s
= 23 + 38
= 62 revolution