Question

A stone is thrown from the top of a building 30m high. If it strikes the ground at an angle 45, with what speed was it thrown?

Answer 1

24.2 m/s

Step-by-step explanation:

The stone strikes the ground at an angle of 45 degrees: this means that its vertical velocity is equal (in magnitude) to its horizontal velocity, in fact:

`tan \theta = (|v_y|)/(v_x)\\tan 45^(\circ) = 1 \rightarrow |v_y| = v_x`

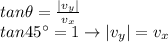
The motion along the vertical direction is a uniformly accelerated motion, so we can find the final vertical velocity using the following suvat equation

`v_y^2 -u_y^2 = 2as`

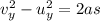
where

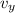`v_y` is the final vertical velocity

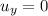`u_y = 0` is the initial vertical velocity (zero because the stone is thrown horizontally)

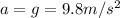`a=g=9.8 m/s^2` is the acceleration of gravity (we take downward as positive direction)

s = 30 m is the vertical displacement

Solving for vy,

`v_y = √(u_y^2+2as)=√(0+2(9.8)(30))=24.2 m/s`

This means that the horizontal velocity is also 24.2 m/s: and since the horizontal velocity is constant during the whole motion (there is no acceleration in the horizontal direction), this means that the stone was thrown exactly at 24.2 m/s.