Question

An investor purchased 100 shares of stock X at \small 6\frac{1}{8} dollars per share and sold them all a year later at 24 dollars per share. If the investor paid a 2 percent brokerage fee on both the total purchase price and the total selling price, which of the following is closest to the investor's percent gain on this investment?(A) 92%

(B) 240%
(C) 280%
(D) 300%
(E) 380%

Answer 1

option (C) 280%

Step-by-step explanation:

Number of shares of stock X purchased = 100

Purchasing cost of share =
`\$6(1)/(8) =(49)/(8)`

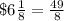
Selling cost of stocks = $24 per share

Brokerage paid = 2%

Now,

The total purchasing cost involved =
`100*(49)/(8)` + 2% of
`100*(49)/(8)`

= 612.5 + 0.02 × 612.5

= $624.75

also,

Total income from sales of stocks

= Total selling cost of shares - brokerage paid

= $24 × 100 - 2% of Total selling cost

= $2400 - ( 0.02 × $2400 )

= $2400 - $48

= $2,352

now,

The investor's percent gain on this investment =
`\frac{\textup{Income-invested amount}}{\textup{Invested amount}}*100\%`

=
`\frac{\textup{2,352 - 624.75}}{\textup{624.75}}*100\%`

=
`\frac{\textup{1727.25}}{\textup{624.75}}*100\%`

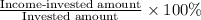
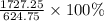
= 276.47% ≈ 280%

Hence, the correct answer is option (C) 280%