Answer:
The maximum compression of the spring is 1.73 cm.
Step-by-step explanation:
Given that,
Mass of box = 5.0 kg
Speed = 6.0 m/s
Spring constant = 60 N/cm
Suppose use the work-energy theorem to find the maximum compression of the spring.
We need to calculate the maximum compression of spring
Using work -energy theorem
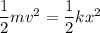

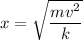
Put the value into the formula
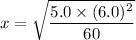
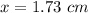
Hence, The maximum compression of the spring is 1.73 cm.