Answer:
see explanation
Explanation:
The equation of a line in slope- intercept form is
y = mx + c ( m is the slope and c the y- intercept )
Rearrange 2x - y - 18 = 0 into this form
Subtract 2x - 18 from both sides
- y = - 2x + 18 ( multiply through by - 1 )
y = 2x - 18 ← in slope- intercept form
with slope m = 2 and y- intercept B(0, - 18)
To find the x- intercept let y = 0 in the equation and solve for x
2x - 18 = 0 ( add 18 to both sides )
2x = 18 ( divide both sides by 2 )
x = 9
Hence x- intercept A(9, 0)
(b)
The perpendicular bisector passes through the midpoint of AB at right angles.
Given a line with slope m then the slope of a line perpendicular to it is
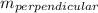 = -
= -
 = -
= -

The midpoint of AB is
[0.5(9 + 0), 0.5(0 - 18) ] = (4.5, - 9)
Thus
y = -
 x + c ← is the partial equation
x + c ← is the partial equation
To find c substitute (4.5, - 9) into the partial equation
- 9 = - 2.25 + c ⇒ c = - 9 + 2.25 = - 6.75 = -
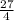
y = -
 x -
x -
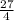 ← in slope- intercept form
← in slope- intercept form
Multiply through by 4
4y = - 2x - 27 ( subtract - 2x - 27 from both sides )
2x + 4y + 27 = 0 ← in general form