Question

Does it pay to ask for a raise? A national survey of heads of households showed the percentage of those who asked for a raise and the percentage who got one. According to the survey, of the women interviewed, 25% had asked for a raise, and of those women who had asked for a raise, 46% received the raise. If a woman is selected at random from the survey population of women, find the following probabilities. (Enter your answers to three decimal places.)

Answer 1

Cross probability

Explanation:

The type of probabilities remains to be determined. That is the question itself. The same problem is found in the book "Understanding basic statistics 7th edition of Brase".

I will assume that the three probabilities to find are:

a) p (woman asked for a raise)

b) p (received raise | asked for one)

c) p (received raise and asked for one)

So,

a) p(woman asked for a raise)

It is given in the statement, so it is simple statistics:

`P_(WomA) =25%`

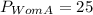
b) p(received raise | asked for one)

It is also found in the statement, however, now the formula would be given by:

p(received raise | asked for one) = p(received raise and asked for one) / p(asked for one)

`P_(WomB)=46%`

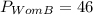
c) p(received raise and asked for one)

For this case the formula is given differently and it is necessary to re-adjust formula B, as follows:

p(received raise and asked for one) = p(received raise | asked for one) * p(asked for one)

So,

`P_(WomC) = 0.46*0.25 = 11.5%`

p(received raise and asked for one) = 11.5%