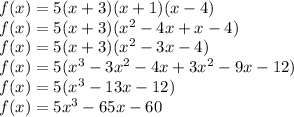Answer:
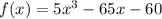
Explanation:
we know that
The roots are
x=-3,x=-4 and x=1
so
The equation of a polynomial of degree 3 with real coefficients and zeros of minus3,minus1, and 4 is equal to
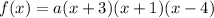
Remember that
 ----> given value
----> given value
For x=-2, f(x)=30
substitute and solve for the coefficient a
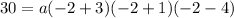
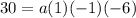
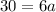

so
The polynomial is
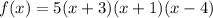
Apply distributive property