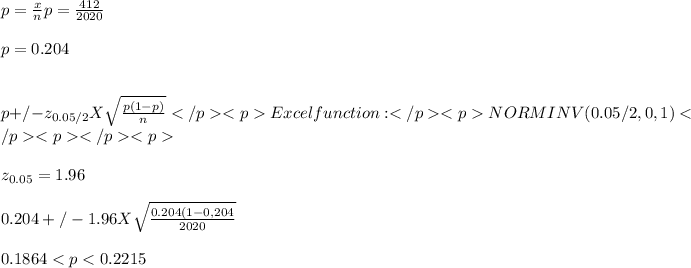
Answer:
0.1864 < p < 0.2215
Step-by-step explanation:
To calculate and construct the 95% confidence interval for the population proportion, it's necessary to use the normal distribution of one sample proportion.
Excel function of normal distribution NORMINV() ---> value of confidence coefficient.