Question

A cartridge electrical heater is shaped as a cylinder of length L=200 mm and outer diameter D=20 mm. Under normal operating conditions the heater dissipates 2 kW while submerged in a water flow that is at 20C and provides a convection heat transfer coefficient of h=5000 W/mK. Neglecting heat transfer from the ends of the heater, determine its surface temperature Ts. If the water flow is inadvertently terminated while the heater continues to operate, the heater surface is ex posed to air that is also at 20C but for which h=50 W/m K. What is the corresponding surface temperature? What are the consequences of such an event?

Answer 1

When water is surrounding T_s = 34.17 degree C

When air surrounding T_S = 1434.7 degree C

from above calculation we can conclude that air is less effective than water as heat transfer agent

Step-by-step explanation:

Given data:

length = 300 mm

Outer diameter = 30 mm

Dissipated energy = 2 kw = 2000 w

Heat transfer coefficient IN WATER = 5000 W/m^2 K

Heat transfer coefficient in air = 50 W/m^2 K

we know that
`q_(convection) =  P`

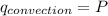
From newton law of coding we have

`q_(convection) =  hA(T_s -  T_(\infity))`

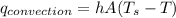
`T_s` is surface temp.

T - temperature at surrounding

`P = hA(T_s -  T_(\infity))</p><p>[tex](P)/(\pi hDL) =  (T_s -  T_(\infity))`

solving for[/tex] T_s [/tex] w have

`T_s = T_(\infty) + (P)/(\pi hDL)`

`T_s = 20 + (2000)/(\pi 5000* 0.03* 0.3)`

`T_s = 34.17 degree C`

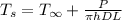
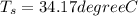
When air is surrounding we have

`T_s = T_(\infty) + (P)/(\pi hDL)`

`T_s = 20 + (2000)/(\pi 2000* 0.03* 0.3)`

`T_s = 1434.7 degree C`

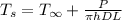
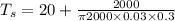
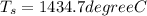
from above calculation we can conclude that air is less effective than water as heat transfer agent