Question

Liquid octane will react with gaseous oxygen to produce gaseous carbon dioxide and gaseous water . Suppose 10.3 g of octane is mixed with 69. g of oxygen. Calculate the maximum mass of carbon dioxide that could be produced by the chemical reaction. Round your answer to significant digits.

Answer 1

`\large \boxed{\text{31.8 g CO}_(2)}`

Step-by-step explanation:

We are given the masses of two reactants and asked to determine the mass of the product.

This looks like a limiting reactant problem.

1. Assemble the information

We will need a balanced equation with masses and molar masses, so let’s gather all the information in one place.

MM: 114.23 32.00 44.01

2C₈H₁₈ + 25O₂ ⟶ 16CO₂ + 18H₂O

Mass/g: 10.3 69.

2. Calculate the moles of each reactant

`\text{Moles of C$_(8)$H$_(18)$} = \text{10.3 g C$_(8)$H$_(18)$} * \frac{\text{1 mol C$_(8)$H$_(18)$}}{\text{114.23 mol C$_(8)$H$_(18)$}} = \text{0.090 17 mol C$_(8)$H$_(18)$}\\\\\text{Moles of O$_(2)$} = \text{69. g O}_(2) * \frac{\text{1 mol O$_(2)$}}{\text{32.00 g O$_(2)$}} = \text{2.16 mol O$_(2)$}`

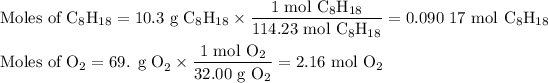
3. Calculate the moles of CO₂ from each reactant

`\textbf{From C$_(8)$H$_(18)$:}\\\text{Moles of CO$_(2)$} =  \text{0.090 17 mol C$_(8)$H$_(18)$} * \frac{\text{16 mol CO$_(2)$}}{\text{2 mol C$_(8)$H$_(18)$}} = \text{0.7214 mol CO}_(2)\\\\\textbf{From O$_(2)$:}\\\text{Moles of CO$_(2)$} =\text{2.16 molO$_(2)$} * \frac{\text{16 mol CO$_(2)$}}{\text{25 mol O$_(2)$}} = \text{1.38 mol CO$_(2)$}\\\\\text{Octane is the limiting reactant because it gives fewer moles of CO$_(2)$.}`

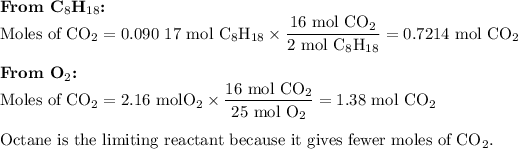
4. Calculate the mass of CO₂

`\text{ Mass of CO$_(2)$} = \text{0.7214 mol CO$_(2)$} * \frac{\text{44.01 g CO$_(2)$}}{\text{1 mol CO$_(2)$}} = \textbf{31.8 g CO}_\mathbf{{2}}\\\\\text{The reaction produces $\large \boxed{\textbf{31.8 g CO}_\mathbf{{2}}}$}`