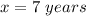Question

2) A $100,000 house appreciates at a rate of $3500 a year.

a. Find the equation that models the value of the house in y dollars after x years.
b. Find the value of the house in 12 years.
c. How many years from now will the value of the house be $124,500?

Answer 1

Part a)
`y=3,500x+100,000`

Part b)
`\$142,000`

Part c)
`7\ years`

Explanation:

Part a) Find the equation that models the value of the house in y dollars after x years

we know that

The linear equation in slope intercept form is equal to

`y=mx+b`

where

m is the rate or slope

b is the y-coordinate of the y-intercept (initial value)

Let

x -----> the number of years

y ----> the value of the house in dollars

In this problem we have

`m=3,500\ (\$)/(year)`

`b=\$100,000`

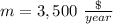
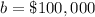
substitute

`y=3,500x+100,000`

Part b) Find the value of the house in 12 years.

so

For x=12 years

substitute in the equation and solve for y

`y=3,500(12)+100,000`

`y=\$142,000`

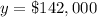
Part c) How many years from now will the value of the house be $124,500?

For y=$124,500

substitute in the equation and solve for x

`124,500=3,500x+100,000`

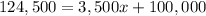
subtract 100,000 both sides

`124,500-100,000=3,500x`

`24,500=3,500x`

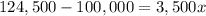
Divide by 3,500 both sides

`x=7\ years`