Answer: a) 453 b) 1537
Explanation:
As per given , we have
Margin of error : E= 0.025
Critical value for 95% confidence interval :
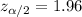
a) The prior estimate of population proportion : p=0.08
Required sample size :-
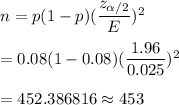
The minimum sample size is 453 U.S. adults.
b) Since the prior estimate of population proportion is not available , so we take p= 0.5
Required sample size :-
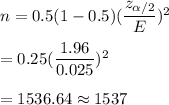
The minimum sample size is 1537 U.S. adults.