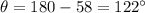Answer:
47.2 units at
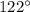
Step-by-step explanation:
The components of the vector are:
x = -25
y = 40
The two components form the sides of a right triangle, of which the vector itself represents the hypothenuse; therefore, we can find the magnitude of the vector by using the Pythagorean theorem:
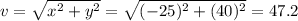
Concerning the direction, we can apply the formula:

The x-component is, however, negative, so the correct angle (measured anticlockwise from the positive x-axis) is