Question

Political Science, Inc. (PSI) specializes in voter polls and surveys designed to keep political office seekers informed of their position in a race. Using telephone surveys, interviewers ask registered voters who they would vote for if the election were held that day. In a recent election campaign, PSI found that 220 registered voters, out of 500 contacted, favored a particular candidate. Develop a 95% confidence interval estimate for the proportion of the population of registered voters that favors the candidate.

Answer 1

[198.421, 241.579]

Explanation:

If 220 registered voters out of 500 contacted favored a particular candidate, we can approximate the mean:

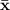`\bf \bar x` = 220

the proportion is

p = 220/500 = 0.44

We can approximate the standard deviation with

`\bf √(500*0.44*(1-0.44))=11.01`

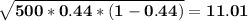
To find a 95% confidence interval estimate for the proportion of the population of registered voters that favors the candidate we must find values a, b such that the area under the Normal curve of mean 220 and standard deviation 11.01 on the interval [a, b] equals 95% = 0.95

We can work out those points with a spreadsheet and find

[a, b] = [198.421, 241.579]

(See picture attached)