Question

An overnight rainstorm has caused a major roadblock. Three massive rocks of mass m1=528 kg, m2=838 kg and m3=311 kg have blocked a busy road. The rocks are side by side by blocking the road and lined up from left to right in order as m1, m2, and m3. The city calls a local contractor to use a bulldozer to clear the road. The bulldozer applies a constant force to m1 to slide the rocks off the road. Assuming the road is a flat frictionless surface and the rocks are all in contact, what force, FA, much be applied to m1 to slowly accelerate the group of rocks from the road at 0.250 m/s^2?FA=___________ NUse the value found above for FA to find the force, F12, exerted by the first rock of mass 528 kg on the middle rock of 838 kg.F12= ___________ N

Answer 1

`FA=419,25N`

`F12=287,25N`

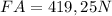
Step-by-step explanation:

The bulldozer is moving the rocks as one system, the weigth for the complete system is:

`M=m1+m2+m3=1677kg`

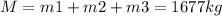
From newton´s second law the acceleration can be related to the force by:

`FA=m*a=1677kg*0,250m/s^(2)=419,25N`

on the middle rock we have the force F12 from the first to the middle rock on the direction of movement and F32 from the las rock to the middle rock on the opposite direction of movement.

For the last rock to accelerate at the same rate it must be subjected to a force:

`F23=m3*a=311kg*0,250m/s^(2) =77,75N`

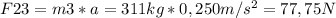
This equals the force F32 on the opposite direction. the resultant force on the middle rock to mantain this acceleration should be:

`F2=m2*a=838kg*0,250m/s^(2) =209,5N`

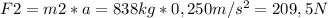
The sum of all the forces applied to the middle rock is:

`F2=F12-F32`

Solving for F12:

`F12=F2+F32=209,5N+77,75N=287,25N`