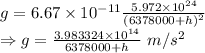Question

The strength of the gravitational field of a source mass can be measured by the magnitude of the acceleration due to gravity at a field point. Thus the gravitational field strength at a point depends on the distance from the source mass. For this problem assume that Earth’s mass is concentrated at its center and that Earth has a radius of RE = 6,378 km at sea level and a mass of ME = 5.972×1024 kg. Ignore any forces due to Earth’s rotation or due to other astronomical bodies.

Answer 1

9.79211 m/s²

Step-by-step explanation:

M = Mass of the Earth = 5.972 × 10²⁴ kg

G = Gravitational constant = 6.67 × 10⁻¹¹ m³/kgs²

r = Radius of Earth = 6378000 m

`g=G(M)/(r^2)\\\Rightarrow g=6.67* 10^(-11)(5.972* 10^(24))/((6378000)^2)\\\Rightarrow g=9.79211\ m/s^2`

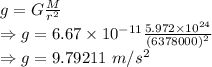
The acceleration due to gravity is 9.79211 m/s²

For any distance above the Earth's surface h

`g=6.67* 10^(-11)(5.972* 10^(24))/((6378000+h)^2)\\\Rightarrow g=(3.983324* 10^(14))/(6378000+h)\ m/s^2`