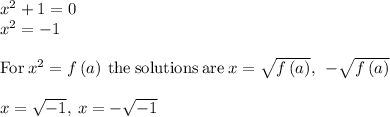Answer:
(a)
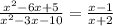 . The domain of this function is all real numbers not equal to -2 or 5.
. The domain of this function is all real numbers not equal to -2 or 5.
(b)
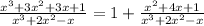 . The domain of this function is all real numbers not equal to 0,
. The domain of this function is all real numbers not equal to 0,
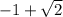 or
or
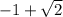 .
.
(c)
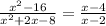 .The domain of this function is all real numbers not equal to 2 or -4.
.The domain of this function is all real numbers not equal to 2 or -4.
(d)
 . The domain of this function is all real numbers not equal to -2.
. The domain of this function is all real numbers not equal to -2.
(e)
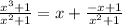 . The domain of this function is all real numbers.
. The domain of this function is all real numbers.
Explanation:
To reduce each rational expression to lowest terms you must:
(a) For
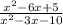
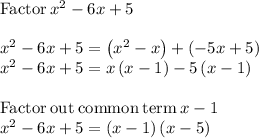
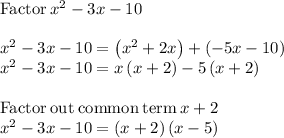
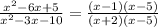
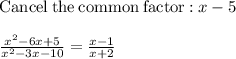
The denominator in a fraction cannot be zero because division by zero is undefined. So we need to figure out what values of the variable(s) in the expression would make the denominator equal zero.
To find any values for x that would make the denominator = 0 you need to set the denominator = 0 and solving the equation.
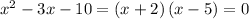
Using the Zero Factor Theorem: = 0 if and only if = 0 or = 0
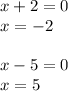
The domain is the set of all possible inputs of a function which allow the function to work. Therefore the domain of this function is all real numbers not equal to -2 or 5.
(b) For
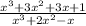
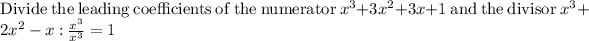
Quotient = 1
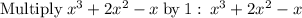
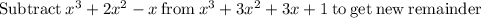
Remainder =
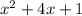
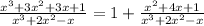
- The domain of this function is all real numbers not equal to 0,
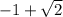 or
or
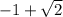 .
.
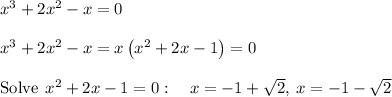
(c) For
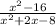
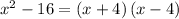

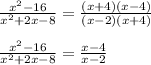
- The domain of this function is all real numbers not equal to 2 or -4.
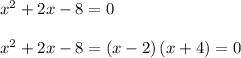
(d) For
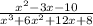
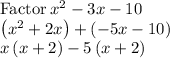
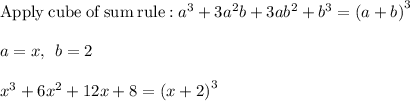
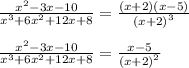
- The domain of this function is all real numbers not equal to -2
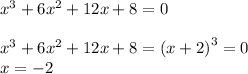
(e) For
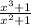
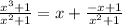
- The domain of this function is all real numbers.