Question

What are the domain and range of f (x) = (one-fifth) Superscript x?

Answer 1

Answer: answer is B

Explanation:

Answer 2

The domain of the function is all real numbers
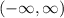`(-\infty,\infty)` and the range is all positive real numbers
`(0,\infty)`

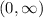
Explanation:

We have the following function
`f(x)=((1)/(5) )^x` and we want to find the domain and the range.

The function we have is an example of an exponential function
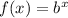`f(x)=b^x` with b > 0 and b ≠ 1. This types of functions in general have the following properties:

• It is always greater than 0, and never crosses the x-axis
• Its domain is the set of real numbers
• Its Range is the Positive Real Numbers
  `(0,\infty)`

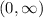
The domain of a function is the specific set of values that the independent variable in a function can take on.

When determining domain it is more convenient to determine where the function would not exist.

This function has no undefined points nor domain constraints. Therefore the domain is
`(-\infty,\infty)`.

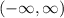The range is the resulting values that the dependent variable can have as x varies throughout the domain. Therefore the range is
`(0,\infty)`.

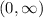We can check our results with the graph of the function.