Question

For the point Pleft parenthesis 5 comma 2 right parenthesis and Qleft parenthesis 12 comma 7 right parenthesis​, find the distance​ d(P,Q) and the coordinates of the midpoint M of the segment PQ.

Answer 1

The distance is
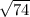`√(74)` which is approximately equal to 8.602 units

The midpoint is (8.5 , 5.5)

Step-by-step explanation:

1- Getting the distance:

The distance between two points can be calculated using the following rule:

`Distance = \sqrt{(x_(2)-x_(1))^2 + (y_(2)-y_(1))^2}`

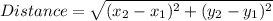
The given points are:

(5,2) represents (x₁ , y₁)

(12,7) represents (x₂ , y₂)

Substitute with the givens in the above equation to get the distance as follows:

`Distance = √((12-5)^2+(7-2)^2)=√(74) = 8.602 units`

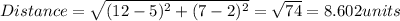
2- Getting the midpoint:

The midpoint of two points is calculated as follows:

`Midpoint = ((x_(1)+x_(2))/(2) , (y_(1)+y_(2))/(2))`

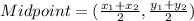
The given points are:

(5,2) represents (x₁ , y₁)

(12,7) represents (x₂ , y₂)

Substitute with the givens in the above equation to get the distance as follows:

`Midpoint = ((5+12)/(2) , (2+7)/(2)) = (8.5 , 4.5)`

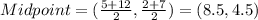
Hope this helps :)