Question

Mohammed has a box of coins containing only dimes

and half-dollars. There are 26 coins, and the total
value is $8.60. How many of each denomination of
coin does he have?

Answer 1

Mohammed has 11 dimes and 15 half-dollars.

Explanation:

Let x be the number of dimes and y be the number of half-dollars. There are 26 coins, then

`x+y=26`

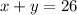
Amount in dimes = 10x cents

Amount in half-dollars = 50y cents

Total amount = 10x + 50y cents

Thus,

`10x+50y=860`

Solve the system of two equations:

`\left\{\begin{array}{l}x+y=26\\10x+50y=860\end{array}\right.`

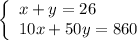
From the first equation

`x=26-y`

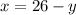
Substitute it into the second equation:

`10(26-y)+50y=860\\ \\26-y+5y=86\\ \\-y+5y=86-26\\ \\4y=60\\ \\y=15\\ \\x=26-15=11`

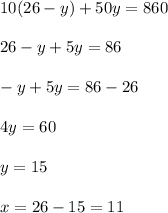
Mohammed has 11 dimes and 15 half-dollars.