Question

What is the equation of the line in standard form? (-3,2) (2,-1)

Answer 1

y=-3/5x+1/5

Explanation:

the picture has a pretty good explanation, but first you do y1 minus y2 over x1 minus x2.

once you get that answer that's your slope.

then plug in one of the ordered pairs for x and y and that will give you b

Answer 2

The equation of the line (-3, 2) and (2, -1) in standard form is
`y=-\left((3)/(5)\right) x+\left((1)/(5)\right)`

Solution:

Given that the points are (-3, 2) and (2, -1)

The equation of the line is given as

`\left(y-y_(1)\right)=m\left(x-x_(1)\right)`

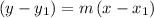
where "m" is the slope of the line and
`x_1 and y_1` are the x and y co-ordinates

The formula of the slope "m" is given as

`m=(\left(x_(2)-x_(1)\right))/(\left(y_(2)-y_(1)\right))`

`m=(-1-2)/(2+3)=(-3)/(5)`

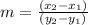
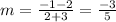
Thus, substituting the above value in the equation of line, we get

`y-2=\left(-(3)/(5)\right)(x+3)`

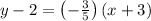
5y - 10 = -3x - 9

5y = -3x + 1

`y=-\left((3)/(5)\right) x+\left((1)/(5)\right)`

Thus the equation of the line (-3, 2) and (2, -1) in standard form is
`y=-\left((3)/(5)\right) x+\left((1)/(5)\right)`