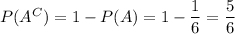Question

Two dice are rolled. Use the complement rule to find that the probability that the sum of the dice is less than 10.

Answer 1

`(5)/(6)`

Explanation:

When two dice are rolled, the sample space is

`\begin{array}{cccccc}(1,1)&(1,2)&(1,3)&(1,4)&(1,5)&(1,6)\\(2,1)&(2,2)&(2,3)&(2,4)&(2,5)&(2,6)\\(3,1)&(3,2)&(3,3)&(3,4)&(3,5)&(3,6)\\(4,1)&(4,2)&(4,3)&(4,4)&(4,5)&(4,6)\\(5,1)&(5,2)&(5,3)&(5,4)&(5,5)&(5,6)\\(6,1)&(6,2)&(6,3)&(6,4)&(6,5)&(6,6)\end{array}`

In total, 36 outcomes

First, find the probability that the sum is 10 or greater:

All favorable outcomes

10:
`(4,6),\ (5,5),\ (6,4)`

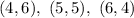
11:
`(5,6),\ (6,5)`

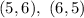
12:
`(6,6)`

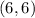
In total, 6 outcomes.

The probability that the sum is 10 or greater is

`P(A)=(6)/(36)=(1)/(6)`

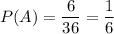
Use the complement rule to find that the probability that the sum of the dice is less than 10:

`P(A^C)=1-P(A)=1-(1)/(6)=(5)/(6)`