Question

Two squares overlap. The area of the overlapped region is 1/4 of the small squares area and is 2/9 of the large square area. What is the ratio of the area of the small square to the large square?

Answer 1

ratio is 8 is to 9

Explanation:

Let small square area be S, and

Large square area be L

We can come up with 2 equations and equate them to find the RATIO.

First, Area of Overlap is 1/4th of small square area. We can write:

A = 1/4*S [A is area of overlapped region]

Second, Area of Overlap is 2/9th of Large square area. We can write:

A = 2/9*L [A is area of overlapped region]

Let's equate both A's to get:

`(1)/(4)S=(2)/(9)L`

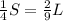
We need ratio of S to L, thus we can rearrange, cross multiply and rearrange to find the ratio of S to L. Shown below:

`(1)/(4)S=(2)/(9)L\\(S)/(4)=(2L)/(9)\\9S=8L\\(9S)/(L)=(8L)/(L)\\(9S)/(L)=8\\(S)/(L)=(8)/(9)`

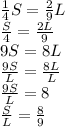
Thus the ratio is 8 is to 9