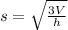1 Answer

Rami Bin Tahin · Answer 1 · 2020-02-09T13:38:57+0000

Answer:

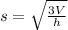
$s=\sqrt{(3V)/(h)}$

Explanation:

We have to re-write the equation and use algebra and solve for s.

This means, we need an expression where it will be "s=SOMETHING".

Lets show the process below:

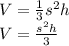
$V=(1)/(3)s^2 h\\V=(s^2 h)/(3)$

Now we cross multiply and solve for s:

$V=(s^2 h)/(3)\\3V=s^2h\\s^2=(3V)/(h)\\s=\sqrt{(3V)/(h)}$

Note: to get s^2 to s, we take square root and eliminate the square

The formula for "s" is:

$s=\sqrt{(3V)/(h)}$

Please log in or register to add a comment.