Answer:
14 cones
Explanation:
First we need to discover the total volume of the wire that we have available to make the cones, so we treat it as a long circular prism, with radios of 1,5 mm and 25,000 milimeters height:
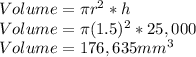
Now we just have to calculate the volume of the cone:
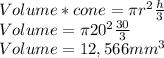
And finally we just have to divide the volume available between the volume necessary for each cone:
176,635/12,566=14.05