Answer:
This is a left-tailed test about a population's mean claim, with known population standard deviation.
c) Claim is alternative, fail to reject the null and cannot support calim as test statistic (-0.89) is not in the rejection region defined by the critical value (-2.05)
Step-by-step explanation:
The claim is alternative because it does not contain an equality. Since we are testing a claim about a population mean when the population standard deviation is known, the statistic in this case is z.
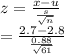
x is the sample mean, u is the population parameter, s is the population standard deviation and n is the number of items sampled.
z = -0.89
The rejection region is going to be the 0.02 (the alfa level) that is to the left of the probability distribution because this is a left-tailed test since the claim is that the population mean is less than 2.8 years. This region is going to be limited by the critical value -2.05.
In a z-core table we can see that for a z = -2.05 the area to the left of the distribution is 0.0202 ≈ 0.02 which is the significance level.