Answer:
Reject
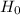
The test statistic is in the rejection region
Explanation:
Since we want to test if the mean predicted by the coach is greater than the mean of the null hypothesis
 , this is a right-tailed test. It means that, in order to reject
, this is a right-tailed test. It means that, in order to reject
 , the test statistic must fall to the right (greater than) of the critical value
, the test statistic must fall to the right (greater than) of the critical value
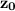
Given that 2.56 > 1.65 then we reject

The test statistic is in the rejection region (greater than 1.65)