Question

Triangle PQR is similar to triangle XYZ. What is the perimeter of triangle XYZ? A. 3in.

B 4 in.
C 12in
D 16in

Answer 1

Perimeter would be C; 12 in.

Answer 2

Answer : The correct option is, (C) 12 in.

Step-by-step explanation :

As we know that when two object have same shape then they are similar. That means, when two triangles are similar then their ratios of lengths of their corresponding sides are equal.

As we are given that triangle PQR is similar to triangle XYZ. That means,

`(PR)/(PQ)=(XZ)/(XY)`

`(12)/(20)=(3)/(XY)`

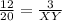
XY = 4 in.

And,

`(PR)/(RQ)=(XZ)/(ZY)`

`(12)/(16)=(3)/(ZY)`

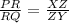
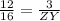
ZY = 5 in.

Now we have to calculate the perimeter of triangle XYZ.

Perimeter of triangle XYZ = Length XY + Length ZY + Length XZ

Perimeter of triangle XYZ = 4 in. + 5 in. + 3 in.

Perimeter of triangle XYZ = 12 in.

Therefore, the perimeter of triangle XYZ is, 12 in.