Missing info in the text: the image is inverted
1) Magnification: -3
The magnification can be calculated with the equation

where
y' is the size of the image
y is the size of the object
In this problem, we have
y' = -60.0 mm (the sign is negative since the image is inverted)
y = 20.0 mm
Substituting,
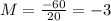
2) Focal length: 0.862 m (converging)
We can also rewrite the magnification as follows
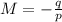 (1)
(1)
where
q is the distance of the image from the mirror
p is the distance of the image from the mirror
Here we know that the distance between the image and the object is 2.30 m, so
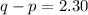
which means
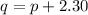
Substituting into (1), we can find p:
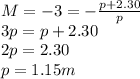
And also q:
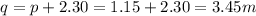
So now we can finally find the focal length by using the lens equation:
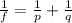
Where f is the focal length. Solving for f,
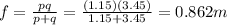
And since the focal length is positive, it means that the mirror is converging.