Answer:
The answer is 3/2.
Explanation:
The rational function is below:
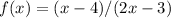
To determine of the vertical asymptote of the rational function we need to find the x value that makes denominator zero.
Then;
2x-3=0
x=3/2
the vertical asymptote for the rational function is 3/2