Question

According to the International Nanny Association (INA), 4,176 nannies were placed in a job last year. (www.nanny.org, 2007). Of course, only 24 were men. In Exercise 3.18 (p. 125) you found the probability that a randomly selected nanny who was placed last year is a man. Now find the probability that in a random sample of 10 nannies who were placed last year, at least 1 is a man

Answer 1

There is a 5.56% probability that there is at one man in this sample

Explanation:

For each nanny, there are only two possible outcomes. Either it is a women, or it is a men. This means that we can solve this problem using the binomial probability distribution.

Binomial probability distribution

The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

`P(X = x) = C_(n,x).\pi^(x).(1-\pi)^(n-x)`

In which
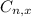`C_(n,x)` is the number of different combinatios of x objects from a set of n elements, given by the following formula.

`C_(n,x) = (n!)/(x!(n-x)!)`

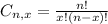
And
`\pi` is the probability of X happening.

Of 4,176 nannies, only 24 were men. This means that
`\pi = (24)/(4176) = 0.0057`.

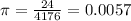Now find the probability that in a random sample of 10 nannies who were placed last year, at least 1 is a man

Sample of 10 nannies, so
`n = 10`

Either there is at least one nanny that is a men, that is probability
`P(X > 0)`, or there are no nannies there are men, that is probability
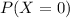`P(X = 0)`. The sum of these probabilities is decimal 1. We want to find
`P(X>0)`.

`P(X > 0) + P(X = 0) = 1`

`P(X > 0) = 1 - P(X = 0)`

`P(X = x) = C_(n,x).\pi^(x).(1-\pi)^(n-x)`

`P(X = 0) = C_(10,0).(0.0057)^(0).(0.9943)^(10) = 0.9444`

`P(X > 0) = 1 - P(X = 0) = 1 - 0.9444 = 0.556`

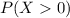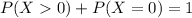
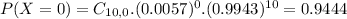
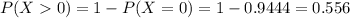
There is a 5.56% probability that there is at one man in this sample