Question

King Waterbeds has an annual cash dividend policy that raises the dividend each year by 44​%. The most recent​ dividend, Div 0Div0​, was $ 0.45$0.45 per share. What is the​ stock's price if a. an investor wants a return of 66​%? b. an investor wants a return of 88​%? c. an investor wants a return of 1111​%? d. an investor wants a return of 1212​%? e. an investor wants a return of 1919​%? a. What is the​ stock's price if an investor wants a return of 66​%?

Answer 1

a.- r= 6% Value: 23.40

`(0.468)/(0.06-0.04) = Intrinsic \: Value`

b.- r = 8% Value: 11.70

`(0.468)/(0.08-0.04) = Intrinsic \: Value`

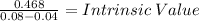
c.- r = 11% Value: 6.69

`(0.468)/(0.11-0.04) = Intrinsic \: Value`

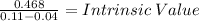
d.- r = 12% Value: 5.85

`(0.468)/(0.12-0.04) = Intrinsic \: Value`

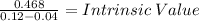
e.- r= 19% Value: 3.12

`(0.468)/(0.19-0.04) = Intrinsic \: Value`

Step-by-step explanation:

We will calcualte the gordon model for the different rates of return:

`(divends_1)/(return-growth) = Intrinsic \: Value`

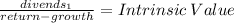
Dividend_1 is next year dividends.

If dividends raise by 4% then:

0.45 x 1.04% = 0.468

now we calculate for the different returns: