Question

Consider the following balanced chemical equation, KCl(aq) AgNO3(aq) → AgCl(s) KNO3(aq)When a sample of impure potassium chloride (0.900 g) was dissolved in water, and treated with excess silver nitrate (AgNO3), 1.26 g of silver chloride (AgCl) was precipitated. Calculate the percentage KCl in the original sample.

Answer 1

In the original sample there's 72.78% KCl

Step-by-step explanation:

KCl(aq) + AgNO₃(aq) → AgCl(s) + KNO₃(aq)

• To solve this problem we need to calculate the mass of pure potasssium chloride. To do that we calculate the moles of KCl that reacted into AgCl:

1.26 g AgCl *
`(1molAgCl)/(143.32gAgCl)*(1molKCl)/(1molAgCl) = 8.792 *10^(-3) molKCl`

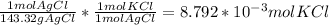
• Now with the molecular weight of KCl, we can calculate the mass of KCl that reacted:

8.792 * 10⁻³ mol KCl * 74.55 g/mol = 0.655 g KCl

• Finally we divide the mass of pure KCl by the mass of the sample, to calculate the percentage KCl:

0.655 / 0.900 * 100% = 72.78%