Question

If a tower is 828m how long will it take to hit the ground

Answer 1

13.5 s

Step-by-step explanation:

An object is dropped from a tower 828 m high. We want to find how long it takes to hit the ground.

The motion of the object is a free fall motion, so we can use the following suvat equation:

`s=ut+(1)/(2)at^2`

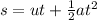
where, taking downward as positive direction:

s = 828 m is the distance covered by the object in its fall

u = 0 is the initial vertical velocity of the object

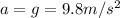`a=g=9.8 m/s^2` is the acceleration of gravity

t is the time taken for the fall

Substituting the numbers and solving for t, we find:

`t=\sqrt{(2s)/(a)}=\sqrt{(2(828))/(9.8)}=13.5 s`