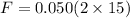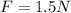Question

Hail comes straight down at a mass rate of m/Δt = 0.050 kg/s and an initial velocity of v0 = -15 m/s and strikes the roof perpendicularly. Unlike rain, hail usually does not come to rest after striking a surface. Instead, the hailstones bounce off the roof of the car. Suppose that the hail bounces off the roof of the car with a velocity of +15 m/s. Ignoring the weight of the hailstones, calculate the force exerted by the hail on the roof.

Answer 1

`F = 1.5 N`

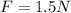
Step-by-step explanation:

Let the hails are coming down with speed "v" and then rebound with the same speed in opposite direction

So here the change in the momentum of the hails is given as

`\Delta P = mv - (-mv)`

`\Delta P = 2mv`

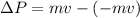
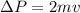
now we know by Newton's 2nd Law

`F = (\Delta P)/(\Delta t)`

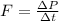
here we have

`F = (\Delta m)/(\Delta t) (2v)`

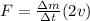
now plug in all data

`F = 0.050(2* 15)`

`F = 1.5 N`